Model for Optimal Regulation for Risk Reduction

As in several of my former posts (1, 2, 3, 4, 5, 6) sometimes I deviate a little from the extreme focus on supply chain risk management, to think about related topics, like today, where I want to highlight the effects of regulation to minimize risks. This article was already written in 1984 by Steven Shavell and it can be downloaded at Harvard University.

Study background

The author, Steven M. Shavell, is a law professor at Harvard law school and associated with the theory of Welfarism.

In his 1984 paper with the title “A model of the optimal use of liability and safety regulation”, he elaborates on the question of how a society should optimally enact legislation to reduce risks on a societal level.

Liability or safety regulation

The model considers accident risks. The two means for reducing this risk, which can be used by legislators, are to a) establish binding safety regulation (e.g. safety standards) or b) establish penalties for liabilities apart from the safety regulation.

Model

Shavell disproves the term “judex non calculat” (judges don’t do math), and establishes a mathematical model with the parameters

1. level of care (x),

1. probability of an accident (p(x)) and

1. the magnitude of harm contingent on the accident happening (h)

Results

The author shows that the level of care with only liability as a measure is in general restricted by the assets of the company and lower then the optimal care (see figure 1).

Figure 1: Level of Care when using only Liability as Risk Mitigation Strategy (Shavell, 1984)

The resulting care under optimal regulation is on the other hand restricted by the likelihood of being caught of non-compliance and the assigned penalties. The comparison of the two measured is displayed in figure 2.

Figure 2: Level of Care when using only Safety Regulation as Risk Mitigation Strategy (Shavell, 1984)

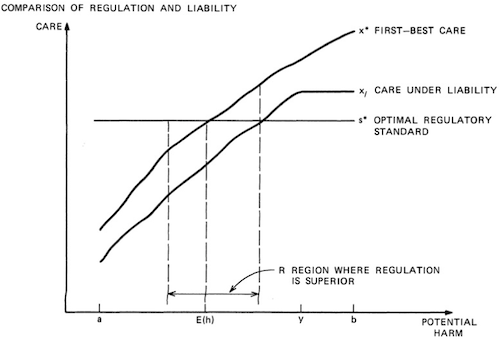
Since both measures do not show optimal results Shavell suggests a combination of both strategies to mitigate the risk and shows that this solution is superior to a single strategy approach in all cases (see figure 3).

Figure 2: Level of Care when using a combination of Safety Regulation and Liability as Risk Mitigation Strategies (Shavell, 1984)

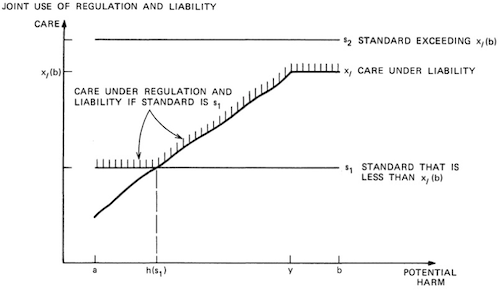
Conclusion

What we see in most societies that there already exists a combination of both measure to reduce the risk of accidents. In most companies liability is still upheld even if current security standards are met. The paper therefore puts emphasis on the point of that both measure should be considered as basis for new legislation.

The paper can also be used to analyze the current state of the art in accident prevention in different legislation systems or industries and deduce findings for further legislation.

Two examples:

• Germany has a huge emphasis on setting security standards, whereas on the other hand corporate liabilities compared to many other countries is fairly minimal.

• Due to their high impact of nuclear catastrophes the nuclear power industry has a risk of high liabilities. This risk on the other hand is restricted by the fact that, if an accident happens the cost often outweigh the assets of the company leading to a default. Therefore sole reliance on the liability mitigation probably does not have a huge effect on the security level. As a result legislation has to emphasis the security standards to achieve an adequate level of care.